Introduction E9F5FC

Questions FFFFC0

• View
• Edit
• History
• Print

Research Program for Math 4 Wisdom

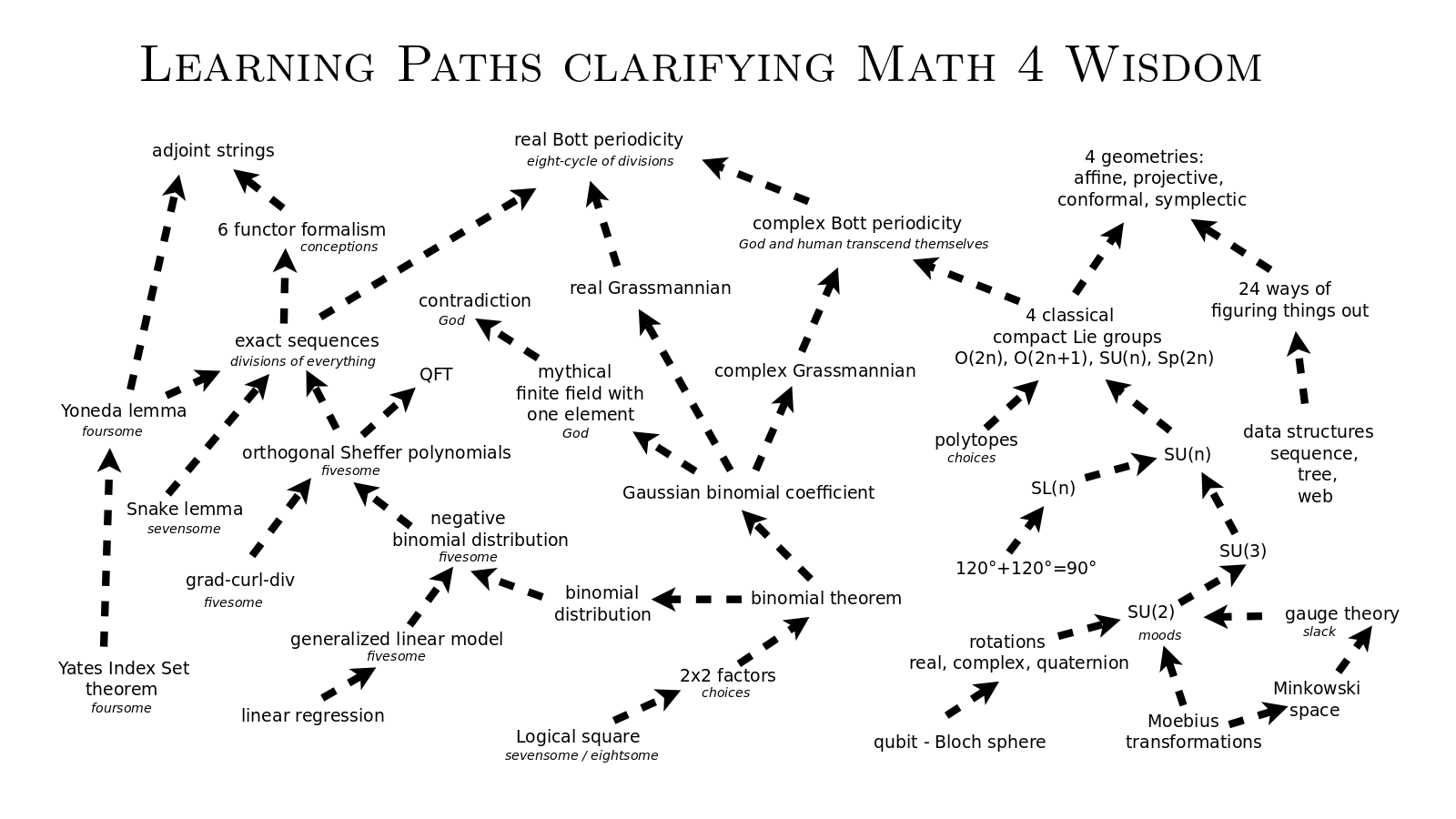
The purpose of Math 4 Wisdom is to show where Wondrous Wisdom arises in advanced mathematics.

A priority is to show how the divisions of everything are expressed by Bott periodicity, Clifford algebras, chain complexes, category theory and other phenomena.

Here are some pages with my recent research.

• Double Causality: A Framework for Physics
• Bott Periodicity Models Consciousness? Preliminary Exploration
• The Yoneda Embedding Expresses Whether, What, How, Why

See: Research priorities for more links

2022.01.19 I will present my research program as straightforwardly as I can. I am working on that below. I am writing text for a series of seven or more videos. I intend to publish my first video by the end of February, 2022. When I have completed the text for that video, I will seek Patreon supporters for my investigations and for my presentations of what I am learning through this website and videos such as a Math 4 Wisdom Show.

In my draft below I am expanding on my 2017 Research Program for a Big Picture of Mathematics.

Mathematically, the key goal is to master Bott periodicity, which relates to seemingly all of the branches of math that I find relevant.

• RP0: Introduction. Research Program.
• RP1: Field With One Element 4 Contradiction 4 God
• Derived functors 4 Threesome 4 Learning
• Yoneda Lemma 4 Foursome 4 Knowledge
• 2-Track Mind or 7-Track Mind for Dualities?
• Quantum Physics 4 Fivesome 4 Deciding
• Snake Lemma 4 Sixsome 4 Morality
• 24 Keys For Solving Math Problems
• Map of Math: Affine, Projective, Conformal, Symplectic Geometry
• Bott Periodicity: Unfolding of Thinking-in-Parallel
• Poincare Group: Organizing Structures and Perspectives
• Big Picture: From Indefinite to Unimaginable

Research to Complete

Before making videos about various parts of my research program, I would like to complete some or all of the relevant research.

Introduction

• Have preliminary thoughts about classifying adjoint strings.
• Understand various examples of adjunction, including for Grothendieck's six operations.
• Understand the induction-restriction adjunction.
• Understand the restriction-coinduction adjunction.
• Understand how the three definitions of adjunction are related.

Threesome

• Understand derived operators.

Foursome

• Understand how Cayley's theorem relates the Yoneda lemma and the induction-restriction adjunction.
• Understand how the Yoneda lemma relates to adjunction.
• Understand how the Yoneda Lemma relates to the Univalence Axiom.

Fivesome

• Be able to generate and calculate Feynman diagrams.
• Accurately state the combinatorics of the Zeng trees and my cycles.

Math Discovery

• Clarify my theory of variables.

Lie theory

• Understand the difference between odd and even dimensional orthogonal groups.

Bott periodicity

• Understand the eightfold nature of the Clifford algebras.
• Understand what are the representations of the Clifford algebras.
• Understand why the Morita equivalence holds for representations of Clifford algebras.

Ask Rimvydas

• What can he tell me about short exact sequences, long exact sequences and derived functors?
  • In particular, what are good examples to think of?
  • What examples would illustrate the three cycle?
  • What examples would illustrate the six-functor formalism? namely, the adjoint functors?
• What can he tell me about the (real and complex) representation theory of Clifford algebras?
  • How to think of Morita equivalence?
  • How to think of eightfold periocity?
• How to think of the difference between odd and even dimensional orthogonal groups?
  • How to think of the difference between affine and projective geometry?
• Can discuss Yoneda Lemma (and its relation to the Univalence Axiom), classification of adjoint strings.
• Can discuss the relation between category theory and homotopy theory.
• Can discuss Schulman's paper.

Overview and Compare Similar Efforts

Most working mathematicians have not spent a few hundred hours in their life searching for a key by which to understand all of mathematics. Indeed, it has been said that Henri Poincare (1854-1912) was the last person to excel at all of the mathematics of his time.

1) One step is to overview the history of mathematics to glean insights from the research interests of the most profound mathematicans, such as Euclid, Descartes, Leibnitz, Pascal, Hilbert, von Neumann and more recently, Weyl, Atiyah, Conway, Grothendieck, Langlands, Lurie to imagine their perspectives on the big picture.

When I was a graduate student (1986-1993), an interest in the big picture was quite taboo, and moreover, quite impractical, given that the way to learn math was to take classes, read textbooks, do exercises, and read journal articles. However, since then, much has changed which has made it possible to learn advanced mathematics much more personally, intuitively, selectively and comprehensively. I can read a vast mathematical encyclopedia (Wikipedia), watch video lectures (You Tube) by expert thinkers on the most advanced subjects, and ask questions and get answers at Math Stack Exchange or Math Overflow.

Of special importance are math bloggers who are sharing their personal intuitions regarding math. It is most strange that intuition is acknowledged as the key to learning and furthering math, and yet articulating, documenting and studying that intuition is considered out of bounds, as can be seen from the little space devoted to it in any article or textbook. The reason, I suppose, is that we would have to reveal our general ignorance. It is particularly refreshing and encouraging to read blog posts by John Baez, Urs Schreiber, Terrence Tao, Qiaucho Yuan and others who do seem to grapple with the big picture.

2) A further step is to note the areas and structures which such bold thinkers believe to be fruitful. Succinctly, as I learn from thinkers such as Olivia Caramello, Urls Schreiber, John Baez, Roger Penrose, Lou Kauffman, Vladimir Voevodsky, Saunders MacLane, William Lawvere, John Isbell, Harvey Friedmann, Joseph Goguen, Robert May, Kirby Urner, Maria Droujkova it seems that they focus on particular areas, such as category theory, topoi, algebraic geometry, homology, homotopy, string theory, network theory but also that they are intrigued by particular structures which seem exceptionally rich, such as the octonions...

Of course, I do not intend to master these subjects in the usual way. Instead, I hope to be clever enough to find a new way of looking at math which shares and yields mathematical intuition much more readily.