- MathNotebook
- MathConcepts
- StudyMath
- Geometry
- Logic
- Bott periodicity
- CategoryTheory
- FieldWithOneElement
- MathDiscovery
- Math Connections
Epistemology
- m a t h 4 w i s d o m - g m a i l
- +370 607 27 665
- My work is in the Public Domain for all to share freely.
- 读物 书 影片 维基百科
Introduction E9F5FC
Questions FFFFC0
Software
Classify adjunctions, Examples of adjunction, Induction restriction adjunction, Adjunction in statistics, Category theory, Limits vs colimits, Equivalence, Sameness, Definition of adjunction, Construction of adjunction, Nonexistence of adjunction, Six functor formalism
Understand the relationship between left adjoints and right adjoints, colimits and limits, and internal structure and external relationships.
伴隨函子
Understanding facts about adjunction
- In what sense is there composition of adjoint functors? So that we can compose the functors from D to C with the functors from C to {$C^J$}, for example?
- How do string diagrams portray adjunctions?
How conditions impact adjunction
- What is a functor that is self-adjoint?
- What are adjoint functors when {$C=D$}?
- What are adjoint functors when {$C\cong D$}?
- What are adjoint functors when F and G are exact inverses?
- Is it possible to have a three-cycle of adjoint endofunctors {$F\dashv G\dashv H\dashv F$} where {$F\cong G\cong H$}?
- What would a three-cycle of functors look like, modeling Penrose's three worlds? Penrose's three-cycle - being, doing, thinking.
Perspectives and divisions
- How do functors express perspectives (or understandings)?
- Is a perspective a morphism between adjoint functors?
- What is the relationship between perspectives-understandings and cones, cocones, limits, colimits, left and right adjunctions?
Relating perspectives
- Are adjoint functors shifts in perspective?
- Do adjunctions express the ways of relating (and transforming) perspectives?
- Where do inverses and approximate inverses come up in my philosophy?
Inversion
- How is adjunction related to the inversion of a functor?
- How is adjunction related to the inversion of a perspective?
Representations
- Are adjoint functors representations?
- Are all adjunctions related to the six functors formalism?
Composition of perspectives
- How do adjoint strings express the composition of perspectives?
- As in "my understanding of her understanding of my understanding" as occurs in adjunctions GF(Y)?
- Express composition of "God's point of view" and "Human's point of view" in terms of adjoint functors.
Category of perspectives
- Is there a category of perspectives?
- Is there an adjunction that models stepping-in and stepping-out?
Divisions
- Do adjoint strings represent divisions?
- In an adjoint string, what are the end points, the "zeroes"?
- What is a functor with no adjoints? In what sense is it the twosome?
Circumstances
- Are there 12 trivialities (the basis for adjunctions) and are they the 12 circumstances-topologies?
Information in context
- What do adjunctions say about knowledge and the foursome?
- If you can't tell the difference between objects, what does knowledge mean?
Levels of knowledge
- How do levels of knowledge correspond to various abilities to distinguish between objects as such?
- Show how the Yoneda Lemma expresses four levels of knowledge - Whether, What, How, Why.
- Understand how the Yoneda embedding and the Yoneda lemma relate to adjunction, for example, how the "do nothing" action appears.
Pairs of levels of knowledge
- Understand how Grothendieck's six operations express these four levels of knowledge (through f) and increasing and decreasing slack (through functors based on an object X).
Extending the domain
- Compare adjoint functor with "extension of the domain", extension of structure.
- How is "extending the domain" related to Kan extensions?
- How are adjunctions and Kan extensions both like extensions of the domain?
- Understand how a Kan extension expresses left and right adjunctions, as in Riehl's book 6.5.2, page 210.
- Try to relate Z and Q and R and other examples to extension of domain, adjunction, Kan extension.
Weakened identity
- Where do isomorphisms, equivalences, dualities fit in? They don't actually contribute structure, context.
Recalibration and communication
- Compare adjoint functor with constructive hypothesis.
- Compare adjoint functor with the pragmatically absolute.
- How does my description of adjunction as expressing communication relate to Shannon theory of information?
- What does Varela mean by complementarity (as in quantum physics between position and momentum)?
- How does the error of recalibration relate to quantum complementarity?
Wholeness preserving function
- What is the relation between Alexander's "wholeness preserving function" and the preservation of limits or colimits?
- How is the triviality of a functor related to wholeness preserving?
Asymmetry of adjoint functors
- In what sense does adjunction express asymmetry?
- Intuit the difference between left adjoints and right adjoints.
- What is the nature of the relationships between right adjoints (and limits) and left adjoints (and colimits)?
- Understand how the qualitative difference between left and right adjoints arises from the asymmetry of how their arrows are related, as shown by the universal mapping property, pointing in parallel or at each other.
- Intuit how the left adjoint exprėsses a local solution and the right adjoint express a global solution. Note that a division of everything relates a particular local perspective within the global overview.
- Adjoint functors describe understanding each other: susikalbėjimas. Where does the asymmetry arise on either side? Is it like a (forgetful) parent and a (free) child understanding each other?
- Think of adjunction in terms of two directions, backwards and forwards, related like the inside and outside of a tube, seeing the same information, but in a different, incomparable context.
From internal structure to external relationships
- How is the internalization of external perspectives (as in the meaning of life) related to the externalization (as relationships) of internal structure?
- How do the 6 ways of proof express structuralization and how does that relate to the distinction between external relationships and internal structure?
Automata
- How does adjunction relate to automata?
- What would be the natural bases for the Yoneda lemma and adjunctions? What space are they describing? What are they indexed by?
Existence of adjoint
- Think of generating the Mandelbrot set in terms of adjoint functors that express {$z\Leftrightarrow z^2+c$}. How would that relate to the Catalan set?
Nonexistence of adjoint
- How does the universal mapping property define the nonexistence of an adjoint?
- What is the relation between K and {$K^{-1}$} in terms of adjunctions? Does the combinatorial interpretation of {$KK^{-1}=I$} (with a minimal local tweak) express that they are left and right adjoints whereas the lack of a combinatorial interpretation for {$K^{-1}K=I$} (no global manipulation) show that they are not right and left adjoints?
- Consider and classify the circumstances under which the adjoint functor does not exist.
Tableaux and {$K^{-1}K=I$}
- Can "the fundamental unit of information" (a certain tableaux-partition) be thought of as an expression of adjoint functors? Consider the duality of matrices and the symmetric group.
- Consider the equation {$K^{-1}K=I$} as a back and forth process.
- Galois theory. Chains express steps of solving. Adjunction. Compare with my involution problem for KK-1=I Existence of chain or not = solvable or not. Relate to the three languages and to learning.
- Use the nonexistence of an adjoint functor to show that there is no combinatorial interpretation of an involution for {$K^{-1}K=I$}.
- Think about Wenbo's infinite factorization of adjunction.
Self-adjoint
- Understand in what sense self-adjoint operators on Hilbert spaces are related to adjunctions.
- Consider how universal enveloping algebras and adjoint representations are related to self-adjoint operators.
- Multiplying a permutation matrix by a column matrix on the right fixes the labels and changes the slots. Multiplying by a row matrix on the left fixes the slots and changes the labels. Can these be thought of as forward and backward views that are related by an adjunction?
- What does it mean for a quantum mechanical operator to be self-adjoint?
Lie theory
- What is the connection between adjoint functors in category theory and adjoint matrices in Lie theory?
- Relate adjunction, adjoint functors with Hermitian adjoint.
- How are inverses, approximate inverses, adjunctions relevant in Lie theory, as in {$UU^*=I$}?
- What is the geometric interpretation of a self-adjoint operator? What does the spectral theorem mean?
- How is multiplying by {$i$} related to adjunction? And complex conjugation?
- How are adjoint functors related to adjunction in Hilbert spaces, as in John Baez's paper?
- In Lie theory, {$UU^*=I$} defines an adjoint representation. Here {$U=U^{-1}$} where we switch the arrows {$i\rightarrow j$} and {$j\rightarrow i$}. And what about the other relationships for arrows? How does this relate to adjunctions and adjoint functors?
- How can a self-adjoint operator or matrix be thought of as an adjoint functor?
- How are self-adjoint (Hermitian) matrices related to unitary matrices?
- How are self-adjoint matrices related to the reals, complexes, quaternions?
- What does diagonalizability, at the heart of self-adjoint operators, mean for adjunctions?
Adjunction and physics
- In the combinatorics of orthogonal polynomials, the polynomials (the information states, what nature has to say as a sum of terms) carries the same information as the space-time weight function (the questions that physicists ask by selecting an interval). Are they related by an adjunction?
- Varela. How does complementarity in physics (momentum and position) (or energy and time) relate to his notion of complementarity as adjunction?
- How to think of the construction of Zeng trees as a free construction (left adjoint)? What is the forgetful right adjoint?
- What would be the adjoint of "availability of free energy"?
Understand
- Awodey, 2006. 9.17: In a sense, every functor has a (right) adjoint! For, given any {$f:C→D$}, we indeed have the right adjoint {$f^∗◦y_D:D→\hat{C}$} except that its values are in the “ideal elements” of the cocompletion {$\hat{C}=Sets^{C^{op}}$}.
- Awodey, 2006. 9.14: Right adjoints preserve limits (remember: “RAPL”!), and left adjoints preserve colimits. Thus if a functor does not preserve limits or colimits, then its corresponding adjoint does not exist.
- Understand the adjoint functor theorem.
- What are "Cellular Automata Evolutions of Adjoints Sequences" of Adenine, Thymine, Guanine, and Cytosine?
- Every right adjoint functor in the category of set is representable. Understand this!
More questions
- Adjunctions and metaphors = topologies & criteria = perspectives on perspectives? = visualizations?
- How are visualizations related to perspectives on perspectives, criteria and topologies?
- Do adjunctions and exact sequences explain how what-> how relates to how->what ?
- In what sense are exact sequences one-directional, asymmetrically, like adjunctions? How are they related to colimits (internal structure) and limits (external structure) ?
- What does adjointness=metaphors mean for relative truth and absolute truth?
- How doe exact strings relate absolute truth (near the zeros as with Whether and Why) and relative truth (in between, as with injection and surjection)?
- Does adjunction reflect the exact sequence?
- Adjoints are organized around a triviality - do they split an exact sequence?
- In what sense do adjoint strings make for a richer metaphor where there is semantic traffic in either direction?
- Conjunction and disjunction, true and false - how are they related to types and adjoints?
读物
- Tom Leinster. Basic Category Theory.
- Lukas Heger. A Brief Introduction to Categories, Part 8: Adjunctions I including Galois connections, Part 9: Adjunctions II With many examples.
- David I. Spivak. Category Theory for Scientists (Old Version)
- MO: What is an intuitive view of adjoints? (category theory)
- Tai-Danae Bradley. What is an Adjunction?, Definition, Examples
- David Ellerman. A Theory of Adjoint Functors--with some Thoughts about their Philosophical Significance. Includes philosophical ideas that seem related to the twosome.
- David Ellerman. Adjoints and emergence: applications of a new theory of adjoint functors.
- Daniel M. Kan. Adjoint Functors
- Bartosz Milewski. Adjunctions.
- Adjunctions are fun. by Kyle Ferendo.
- Keegan Smith. Adjoint Functors in Algebra, Topology and Mathematical Logic.
- Fausk, Hu, May. Isomorphisms Between Left and Right Adjoints
- Tai-Danae Bradley, Tyler Bryson, and John Terilla. Topology: A Categorical Approach dedicates a lot of attention to adjoint functors.
- John Baez. Lectures on advanced category theory
- David Ellerman. A Theory of Adjoint Functors philosophy of communication
- 科学期刊文章: David Ellerman. Adjoints and Emergence: Applications of a new theory of adjoint functors.
- 科学期刊文章: David Ellerman. Mac Lane, Bourbaki, and Adjoints: A Heteromorphic Retrospective
- Louis Kauffman. Mathematical Work of Francisco Varela.
- Goguen, Varela. Systems and Distinctions. Duality and Complementarity.
- Adjunction and inversion of adjunction. Adjunction formula. We call adjunction the process of inferring statements about a subvariety from some knowledge of the ambient variety, while the inverse and usually more complicated process is called inversion of adjunction.
- https://www.researchgate.net/publication/51978740_Relating_Operator_Spaces_via_Adjunctions
- https://hal.archives-ouvertes.fr/hal-02184208/document Crash Course on Category Theory focusing on adjunctions.
- Chris Henderson. Generalized Abstract Nonsense: Category Theory and Adjunctions Equivalence of two definitions.
- Michael Levin. Agency at the Very Bottom.
维基百科
- Wikipedia: Adjoint functors
- Wikipedia: Funtores adjuntos
- Wikipedia: Adjunktion
- Wikipedia: Foncteur adjoint
- Сопряжённые функторы
- 伴隨函子
- Galois connection
- Monad
- Kuratowski closure axioms
- 维基百科: Adjoint Notions of adjoint.
- Adjunction formula Relates the canonical bundle of a variety and a hypersurface inside that variety.
影片
- 影片: Richard Southwell: Adjoint Functors
- Catsters: Adjunctions from morphisms Motivation for the construction of adjoint functors for bundles over sets.
- Emily Riehl. The Formal Theory of Adjunctions Monads Algebras and Descent
- Chris Rogers. Towards an adjunction between the homotopy theories of dg manifolds and Lie ∞-groupoids
- MathProofsable. Adjunction.
Software
- https://varkor.github.io/blog/2020/11/25/announcing-quiver.html Quiver - for drawing commutative diagrams
事实 (Shìshí)
- Uniqueness Two right adjoints of a functor are naturally isomorphic.
直觉 (Zhíjué)
Intuition about adjunction
Meaning of adjunction: Analogy
- Given two worlds (such as {$C=\mathbf{Set}$} and {$D=\mathbf{VectorSpaces}$}) and a functor {$F:C\rightarrow D$} (metaphor) between the two worlds.
- Then the left adjoint is the functor L which defines an analogy between arrows {$L(d)\rightarrow c$} in the world {$C$} and arrows {$d\rightarrow F(c)$} in the world {$D$}.
- And the right adjoint is the functor R which defines an analogy between arrows {$F(c)\rightarrow d$} in the world {$D$} and arrows {$c\rightarrow R(d)$} in the world {$C$}.
- In either case the definition depends on it holding for all {$c$} in {$C$} and all {$d$} in {$D$}. And if it holds, then it is unique up to isomorphism.
- From the analogy it is clear that the left adjoint acts internally as it prepares (preprocesses) the input, as with colimits, whereas the right adjoint acts externally as it processes (postprocesses) the output, as with limits. Indeed, this distinction between colimits and limits is manifest in the adjunctions that arise from the diagonal functors (copying an object into diagrams).
- For example, given the diagonal functor {$\Delta:c\rightarrow (c,c)$}, the left adjoint has to handle the information of {$X$} and {$Y$} by preprocessing, thus internally establishing the coproduct, whereas the right adjoint handles the information by postprocessing, thus by externally establishing the product.
- A functor F with both a left adjoint and a right adjoint plays both roles - preprocessing (with regard to its right adjoint) and postprocessing (with regard to its left adjoint)
- Michael Levin on adjunctions and active inference: Optimal reconstruction (relates to internal modeling of external environment). Laziest way to do something (relates to least action). Prediction is structure preserving transformations. Math is agentic because it makes prediction.
Meaning of adjunction: Metaphor
- Adjointness = metaphors. Metaphors is layered. There are layers of metaphors.
- Meanings are variously related by adjunctions. They enrich the meaning and extend the context.
- Think of my understanding of my three grandfathers as changing with context.
Information in context
- If you wrote {$U=\textrm{Res}^G_H W$} then you would not go back. Except that the knowledge is not in this representation but in the map that takes you back up. So it is outside knowlege, this implicit knowledge.
- Extending context. Adjunction is like extension of the domain, but in terms of structure: extension of structure. Polymorphism.
- Adjunctions present the same information in different context. Information is given in the form of morphisms, not isolated objects. Morphisms are structure preserving maps, or more generally, validations that there is a way to go from one object to another.
- An adjunction relates contexts (categories) that are connected by patches (images of functors). Which is to say, an adjunction synchronizes two categories along a patch that may be all or part of the category on either side.
- Adjoint functors harmonize the semantics of information (which stays the same) and the syntax of context (which changes).
- A morphism synchronizes the clockwork of one representation with the clockwork of another representation. This synchronization is information. An adjunction matches this synchronization in one world (for one group) with the synchronization in another world (for another group). The matching is a natural isomorphism.
From internal structure to external relationships
- Adjunction relates a question (external relationships, what we don't know) and an answer (internal structure, what we know). External relationships are a universal language of questions, of what we don't know.
- Those things are which show themselves to be. This means that internal structure must get expressed through external relationships, as with category theory. Colimits are internal structures and limits are external relationships. Thus colimits need to get expressed as limits. A topos has arbitrary colimits and finite limits, just as a topology has arbitrary unions and finite intersections. Right adjoint functors preserve limits and left adjoint functors preserve colimits. Thus we should move from left adjoint functors (such as adding perspectives, as with free constructions) to right adjoint functors (removing perspectives, as with forgetting).
- Those things are which show themselves to be. Shulman: By analogy with function extensionality and propositional extensionality, univalence could be called typal extensionality. In particular, like function extensionality and propositional extensionality, univalence is an “extensionality” property, meaning that “types are determined by their behavior.”
Perspective
- A functor is a perspective. An adjoint functor is an inverted perspective.
- David Ellerman. On Adjoint and Brain Functors. A heterodox treatment of adjoints using heteromorphisms (object-to-object morphisms between objects of di§erent categories) that parses an adjunction into two separate parts (left and right representations of heteromorphisms). Then these separate parts can be recombined in a new way to define a cognate concept, the brain functor, to abstractly model the functions of perception and action of a brain.
- Adjoint functors relate human and divine perspectives in trivial ways.
- Adjoint functors may relate to chains of perspectives of human's view and God's view. The identity triangles for units and counits are in terms of FGF. This reminds me of my thoughts on bisecting a view. FGF also shows the ambiguity of F, that it can be thought of as acting on the outside, F(GF->I), or on the inside (FG->I)_F
A perspective in a division of everything may be not a functor but an adjunction between functors.
- Wall = nullsome
- Wall - Wall = onesome
- Wall - A - Wall = twosome
- Wall - A - B - Wall = thresome (adjunction)
- Wall - A - B - C - Wall = foursome (adjoint string of length 3)
Forwards view and backwards view
- Adjunction is like a half-inverted tube. There is an inversion point which can be changed, which can be freely chosen as desired, but cannot be either end.
- Adjoint functors relate viewing forward and viewing backward as occurs with shifts of perspective in the foursome, fivesome, sixsome, sevensome.
Pushdown automata
- Adjunction's left and right parentheses are like particle and anti-particle, which can be created out of an identity morphism by way of the triangle identity.
- Adjunction's parenthesis in one world mirrors an identity in the other world.
- Adjunction's parentheses (unit and counit) and their respective worlds express reading and writing.
- Adjunction's parenthesis (unit or counit) is the boundary of a world (into or out of the image of the functor).
Approximate inverse functor from above or below
- An adjoint functor is an approximate inverse, either as a pre-adjustment ("on the left") or as a post-adjustment ("on the right").
Left or right
- In adjoint strings, each functor changes from being left adjoint or right adjoint. So it's not possible to directly compare the different homset isomorphisms because the homsets for left and right are not the same.
Ambiguity
- Adjunction includes shared ambiguity inside of the bijection. For example, in the tensor product - homset adjunction, the functors differ as to how they treat y, but they treat x basically the same and likewise they treat z basically the same. In the induction-restriction adjunction there is a great ambiguity as to how to interpret the action on H but they differ on G, where one is defined and the other is not.
Definition of adjunction
- An adjunction is defined by two different natural transformations that are separated by a functor (before and after) and a related functor (after and before).
Classifying trivial cognition
- Adjunction gives the kinds of dualities and trivialities.
- Adjunctions are trivial nonsymmetries.
Wholeness transformation
- An adjoint functor, notably a trivial one, is a wholeness transformation, as described by Christopher Alexander.
Emphasis of morphisms
- Adjunction is a relationship that lifts above the object and says what matters are the morphisms. But it does that with a pair of counterbalancing functors.
Homotopy equivalences
- How algebraic topology and category theory are related. Adjunctions are homotopy equivalences.
Recalibration
- Adjunction: G(F(x)) is the recalibration of x
Explosion of unfolding
- Mathematical explosion can be based on adjunction explosion as when going back and forth between geometry and algebra, each becoming more refined and powerful
Restructuring
- Adjunction can describe the details of restructuring of one structure by another structure and the abyss between them.
Weakening of identity
- Adjunction example: Lending a dollar bill and not getting back the same dollar bill, but an equivalent dollar bill.
- Equivalence - how identity appears on the inside.
- Isomorphism - how identity appears on the outside.
- C and D are equivalent (there are natural isomorphisms from {$FG$} to {$I_D$} and {$I_C$} to {$GF$}) if and only if {$F\dashv G$} and both F and G are full and faithful. More generally, an adjunction describes the ways in which F and G may not be full and faithful. A functor {$F:C\rightarrow D$} induces a function {$F_{X,Y}:\textrm{Hom}_C(X,Y)\rightarrow\textrm{Hom}_D(F(X),F(Y))$}. {$F$} is faithful if {$F_{X,Y}$} is injective and is full if {$F_{X,Y}$} is surjective. So there are four possibilities for F: It is both injective and surjective, only injective, only surjective, and neither. Likewise there are four possiblities for G. Thus there are {$4\times 4=16$} possibilities in all to explore.
Adjunctions and perspectives and divisions
Divisions
- If you can have two functors that are both left and right adjoints of each other, then they can form adjoint strings of arbitrary length, thus express divisions of everything of arbitrary length. An example is the case of induction - restriction - coinduction. This brings to mind the reflection effect for the twosome, by which the direction can change, if you reflect.
Twosome
- The adjunction of free construction (free will) and forgetful (fate) functors expresses the twosome.
- The twosome is modeled by the adjunction of the tensor product functor (opposites coexist - in parallel - commutative, internal duality A=A) and the homset functor (all is the same - in series - noncommutative, external duality A->B).
- Varela. 10.6 Excursus into Dialects. Examples related to the twosome and threesome.
Adjunction - shifts
- +1 self-adjoint operator
- +2 restriction-induction (implicit information - internal structure vs. explicit information - external relationships)
- +3 three-cycle derived functors
Operation +2
- Adjoint strings express the operation +2, the alternation of Human perspective of God's perspective, and also thus the gap that starts with the foursome, grows with the fivesome, sixsome, sevensome, where from that womb keep inserting new perspectives, flipping the direction, what it means to go beyond oneself.
Six functors formalism. Six representatives.
- {$C\rightarrow C$} 2: tensor & homset
- {$C\rightarrow \textrm{Set}$} 4: sheaves
Left and right derived functors
- Left and right derived functors. A main interest of homological algebra is to study functors between categories of modules (or more generally abelian categories) via the homology of their compositions with other functors. One of the crucial tools for doing so is the concept of derived functors. The categories in question must have projective or injective objects, and enough of them at that, for this idea to work. The functor to be studied is then applied to projective or injective resolutions, chain complexes consisting of projective/injective objects, after which homology is taken. [Wei94, § 2.1–§ 2.5], [Bla11, § 11.2–§ 11.3]
- 21. Derived functors/categories (e.g., Tor, Ext, triangulated categories) The topics no. 17, “Homological algebra”, and no. 21, “Derived functors/categories (e.g., Tor, Ext, triangulated categories)”, are for all intents and purposes identical. Having multiple talks on it seems very much possible. Up to four are outlined below. Chain complexes. Chain complexes, usually of modules over a ring, are the prominent entities treated in homological algebra. Chain complexes form a category with chain maps as morphisms. Actually, they form a 2-category in the sense of higher order category theory with chain homotopies as second-order morphisms. The functor given by the operation of taking the homology of given chain complex is what gives the subject of homological algebra its name. It permits measuring to what degree a given chain complex fails to be exact (a property inspired by that of the same name for short or long sequences). [Wei94, § 1.1–§ 1.5], [Bla11, § 11.1]
- Triangulated categories. The theory of derived functors can alternatively be expressed through that of derived categories, a special case of triangulated categories (or more precisely triangulated categories with chosen t-structures). Generalizing abelian categories, triangulated categories are merely additive categories but come equipped with a generalized notion of “exact sequences”, namely exact triangles. Much of classical homological algebra can be carried out on triangulated categories as well. [Wei94, § 10.1–§ 10.2]
Additional topics
Proof of nonexistence of adjoint
{$K^{-1}K=I$}.
- Partitions (tableaux) are structures that relate to decomposition (factoring) of an item. Thus they relate to factorization of monoids. Thus it may be possible to show using adjunctions that they can't yield a graded involution, one that factors through.
- My thesis about eigenvalues of a matrix may involve an adjunction that relates a substitution right adjoint functor (for the matrix entries) and a left adjoint functor for reworking of nodes into edges.
Adjunctions and Math discovery
- Tensor-hom is like a list
- Galois connections are like least upper bound, greatest lower bound
- Diagonal functor is like substitution (one of the methods of proof)
- In the house of knowledge, the threesome creates a division of everything between local (algebra) and global (analysis) as an adjoint string.
Self-adjointness
- Hermitian adjoint The adjoint of an operator plays the role of the complex conjugate of a complex number.
- Self-adjoint operators play the role of the real numbers (being equal to their own "complex conjugate") and form a real vector space. They serve as the model of real-valued observables in quantum mechanics.
- The relation between self-adjoint (reals) and adjoint (complexes) gets repeated with the complexes and the quaternions, and with the quaternions and the octonions.
- If we think of the scalar product as summing over all elements of the group and thus ensuring group invariance, and if that scalar product is semilinear in the second argument, then inverting all of the group elements is the same as transposing and taking the complex conjugate of the matrix. Thus adjunction expresses this duality.
- If a representation is defined with regard to an orthonormal basis, then it is a unitary matrix. We can think of a unitary matrix as expressing that a representation is defined with regard to an orthonormal basis defined with regard to the scalar product.
- Self-adjoint means that it is "observable" because observability requires being able to approach it from both directions of causality, forwards and backwards, as per the critical point - the ambiguous point - of the fivesome.
- A unitary (self-adjoint) transformation has the same information going forward as going backward (there is an adjunction) but that information may be shifted (as when shifting an infinite dimensional vector space). Equality after a shift is what is possible (expresses the freedom) in a closed system, as modeled by mathematics, where you have self superimposed sequence (an internal, analytic symmetry).
Relating adjunctions in category theory and linear algebra
- In linear/Lie algebra - Hermitian adjoint (Hermitian conjugate) - {$<Ah_1,h_2>H_2=<h_1,A*h_2>H_1$}
- Self-adjoint operator: {$A**=A (A*)-1=(A-1)*$}
Adjunction in physics
- Free construction moves us from kinetic energy to potential energy. Forgetting moves us from potential energy to kinetic energy.
- The change in the free energy is the maximum amount of work that a thermodynamic system can perform in a process at constant temperature, and its sign indicates whether a process is thermodynamically favorable or forbidden. Free energy is subject to irreversible loss in the course of such work.
- A propagator {$<u_n(x'),u_n(x)e^{-iE_nt/ħ}>$} relates a shift from {$x$} to {$x'$} with multiplying by a time factor.
Adjunction and physical operators
- Information states: What nature has to say as a sum of terms.
- Space-time weight function: What questions the physicists ask by selecting an interval.
Correlation and causality
- Adjunction can relate correlation and causality. For they are two functors that are synchronized but they have different contexts. A correlation is like a forgetful functor in that it gives partial information. And causality is like a free construction in that it generates more information.
Emergence
- Mathematically, emergence is described by free construction, thus left adjoints.
Adjunction and entropy
- Information context. Probability. The value of the change in a bit.
- Local information is high entropy (Shannon commmunication). Global informaion is low entropy (Physicists).
- von Neumann entanglement entropy - system (there is a composite state) and subsystem (there is no state).
Wisdom
Unfolding vs. Void
| Left adjoint | Right adjoint | Notes |
| Eternal life | God | What about good? and life? |
| Composition | Identity morphism |
Organization of knowledge
Notes: Lecture 15: Abstraction and adjunction, video by Daniel Murfet
- Seminar: How to use adjoint functors and topoi to organise mathematical knowledge, Announcement
- Based on book: Mac Lane, Moerdijk. “Sheaves in Geometry and Logic: A First Introduction to Topos Theory”.
- See:
 Classifying topos and
Classifying topos and  Classifying space
Classifying space

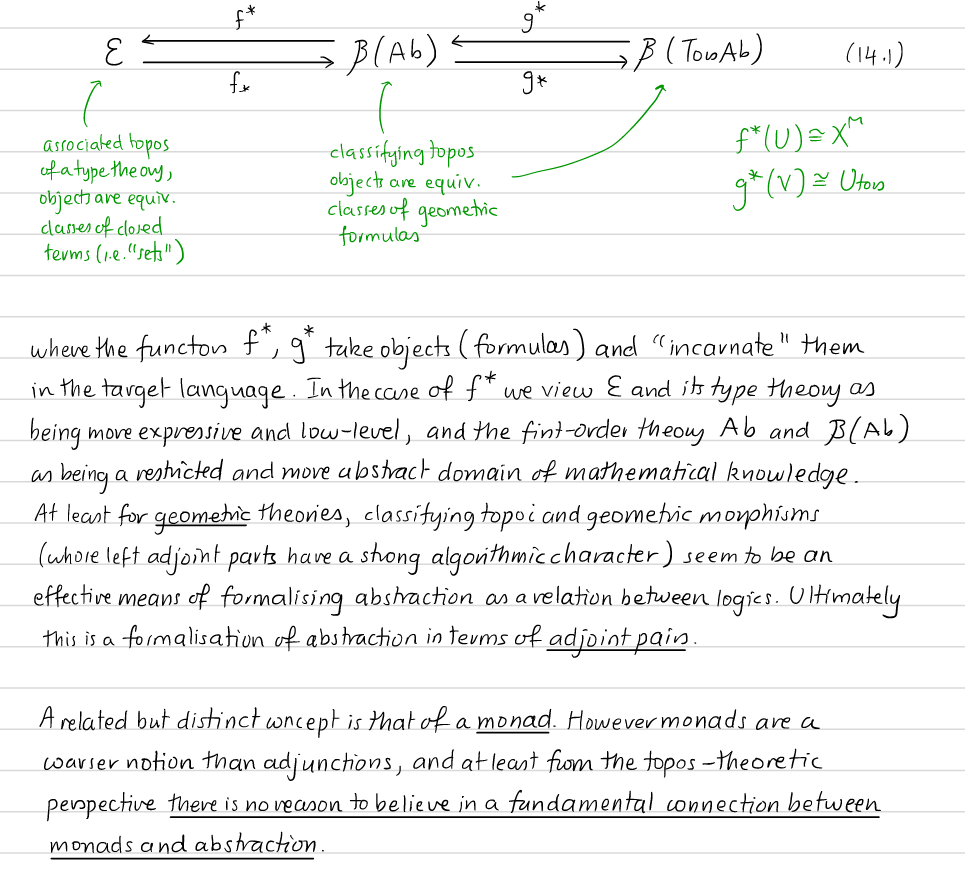

Notes
- For the Geometry of Moods video I could have added one more slide about Conformal Geometry, SL(2) and the threesome.
- Monads deal with scopes: none, some, and so on. The logic of the sevensome.
- Eugenio Moggi. Notions of computation and monads
- Anurag Mendhekar. So, You Want To Understand Monads?
- An Intuitive Introduction to Monads in Under 10 Minutes Polymorphic embellished composition. Defined for overloads. Monads are generic containers whose prototype can be overridden by its usage in the program and implicitly declares "exceptional" behavior, including side effects that would otherwise not be possible to have in a purely functional programming language, and hides rules for handling such behavior inside of itself.
- Relate triangulated categories (with squiggles {$X\rightsquigarrow W = X\rightarrow TW$}) to monads with likewise squiggles.
- Adjoint School 2023
