• View
• Edit
• History
• Print

Meixner polynomials

Recurrence relation

T. S. Chihara, An Introduction to Orthogonal Polynomials, Gordon and Breach, New York, 1978.

Chihara defines the Meixner polynomials as follows. They satisfy the recurrence relation for orthogonal Sheffer polynomials:

{$P_{n+1}(x)=[x - (dn + f)]P_n(x) - [n(gn + h)]P_{n-1}(x)$} where {$g>0, g+h>0, d, f\in\mathbb{R}, d^2-4g\neq 0$}

{$d^2-4g >0$} yields the Meixner polynomials and {$d^2-4g <0$} yields the Meixner-Pollaczek polynomials. Here we focus on the Meixner polynomials.

Chihara expresses {$c,\rho,\beta$} in terms of the variables above:

• {$c=\frac{d-\rho}{d+\rho}$}
• {$\rho = \sqrt{d^2-4g}$}
• {$\beta = 1+\frac{h}{g}$}

We can rewrite {$d, f, g, h$} in terms of {$c,\rho,\beta$} as follows:

• {$d=\rho\frac{1+c}{1-c}$}
• {$f=\beta\rho\frac{c}{1-c}$}
• {$g=\rho^2\frac{c}{(1-c)^2}$}
• {$h=(\beta -1)\rho^2\frac{c}{(1-c)^2}$}

We can thus rewrite the recurrence relation (for orthogonal Sheffer polynomials) as follows:

• Chihara: {$P_{n+1}(x)=[x - \rho\frac{c}{1-c}(n (1+\frac{1}{c})+\beta)]P_n(x) - n\rho^2\frac{c}{(1-c)^2}[n -1 + \beta ]P_{n-1}(x)$}
• Kim and Zeng: {$P_{n+1}(x)=[x-(\alpha\beta + nu_3+nu_4)]P_n(x)- u_1u_2 n(n-1 +\beta )P_{n-1}(x)$}
• Kim and Zeng: {$P_{n+1}(x)=[x - (u_3+u_4)n + \alpha\beta)]P_n(x)-[u_1u_2n(n-1) + u_1u_2\beta n]P_{n-1}(x)$}
• Andrius: {$P_{n+1}(x)=[x-(ln+cf)]P_n(x)-[kn(n-1) + nc]P_{n-1}(x)$}
• Andrius: {$P_{n+1}(x)=[x-(((-\alpha)+(-\beta))n+cf)]P_n(x)-(-\alpha)(-\beta)[n(n-1) + nc]P_{n-1}(x)$}

Thus we can express Chihara's variables in terms of my variables:

• {$d=-\alpha -\beta, g=(-\alpha)(-\beta), h=(c -1)(-\alpha)(-\beta)$}
• and thus {$\rho=\sqrt{d^2-4g}= |\alpha - \beta|$}
• {$c_{Ch}=\frac{\alpha}{\beta}$} if {$\alpha > \beta$} and {$|c|>1 \Leftrightarrow |\alpha|>|\beta|$}, and analogously, {$c_{Ch}=\frac{\beta}{\alpha}$} if {$\beta > \alpha$} and {$|c|>1\Leftrightarrow |\beta|>|\alpha|$}. Furthermore {$c<0 \Leftrightarrow \alpha\beta < 0$}.
• {$\rho\frac{c}{1-c}(1+\frac{1}{c})=\rho\frac{1+c}{1-c}=-\alpha -\beta$}
• {$\rho^2\frac{c}{(1-c)^2} = (-\alpha)(-\beta)$}

Likewise, my variables can be expressed in terms of Chihara's variables. Solving the last two equations:

• {$\alpha_A = \frac{c\rho}{c-1}$}
• {$\beta_A = \frac{\rho}{c-1}$}
• {$c_A=\beta$}
• {$f_A=\frac{f}{\beta}$}

We can express Kim and Zeng's variables in terms of my variables, and vice versa: {$\alpha_{KZ}=f_A, \beta_{KZ}=c_A, u_{1-KZ}=u_{4-KZ}=-\alpha_A, u_{2-KZ}=u_{3-KZ}=-\beta_A$}

Then Meixner polynomials are defined as

• Chihara: {$m_n(x;\beta,c)=(\frac{c-1}{cp})^nP_n(\rho x)$}
• Andrius: {$m_n(x;c,\frac{\alpha}{\beta})=\frac{1}{\alpha^n}P_n((\alpha-\beta)x)$}.

We derive the recurrence relation for the Meixner polynomials as given by Chihara:

• {$P_{n+1}(\rho x)=[\rho x - \rho\frac{c}{1-c}(n(1+\frac{1}{c})+\beta)]P_n(\rho x) - n\rho^2\frac{c}{(1-c)^2}[n-1+\beta]P_{n-1}(\rho x)$}
• {$(\frac{c-1}{c\rho})^{n+1}P_{n+1}(\rho x)=(\frac{c-1}{c\rho})^{n+1}[\rho x - \rho\frac{c}{1-c}(n(1+\frac{1}{c})+\beta)]P_n(\rho x) - (\frac{c-1}{c\rho})^{n+1}n\rho^2\frac{c}{(1-c)^2}[n-1+\beta]P_{n-1}(\rho x)$}
• {$m_{n+1}(x)=(\frac{c-1}{c\rho})[\rho x - \rho\frac{c}{1-c}(n(1+\frac{1}{c})+\beta)]m_n(x) - (\frac{c-1}{c\rho})^2 n\rho^2\frac{c}{(1-c)^2}[n-1+\beta]m_{n-1}(x)$}
• {$m_{n+1}(x)=(\frac{c-1}{c})[x - \frac{c}{1-c}(n(1+\frac{1}{c})+\beta)]m_n(x) - \frac{n}{c}[n-1+\beta]m_{n-1}(x)$}
• {$cm_{n+1}(x)=[(c-1)x + (1+c)n + c\beta]m_n(x)-n(n-1+\beta)m_{n-1}(x)$}
• Multiplying by {$\frac{1}{c}$} yields the relation given by Anne de Medicis: {$m_{n+1}(x)=((1-\frac{1}{c})x + (1+\frac{1}{c})n + \beta)m_n(x)-\frac{n}{c}(n+\beta -1)m_{n-1}(x;\beta,c)$}

In my variables, this becomes

• {$P_{n+1}((\alpha -\beta)x)=[(\alpha -\beta)x - ((-\alpha)+(-\beta))n - \alpha c)]P_n((\alpha -\beta) x) - (-\alpha)(-\beta)[n(n-1) + nc]P_{n-1}((\alpha -\beta) x)$}
• {$\frac{1}{\alpha^{n+1}}P_{n+1}((\alpha -\beta)x)=\frac{1}{\alpha^{n+1}}[(\alpha -\beta)x - ((-\alpha)+(-\beta))n -\alpha c)]P_n((\alpha -\beta) x) - \frac{1}{\alpha^{n+1}}(-\alpha)(-\beta)[n(n-1) + nc]P_{n-1}((\alpha -\beta) x)$}
• {$m_{n+1}(x)=\frac{1}{\alpha}[(\alpha -\beta)x - ((-\alpha)+(-\beta))n-\alpha c)]m_n(x) - \frac{1}{\alpha^2}(-\alpha)(-\beta)[n(n-1) + nc]m_{n-1}(x)$}
• {$\frac{\alpha}{\beta}m_{n+1}(x)=[\frac{\alpha -\beta}{\beta}x + \frac{\alpha + \beta}{\beta}n+\frac{\alpha}{\beta}c]m_n(x) - [n(n-1) + nc]m_{n-1}(x)$}

Which accords with Chihara's equation. And we can write this as follows to emphasize the distinction between traveling steps {$\alpha$} in one direction and traveling steps {$\beta$} in the opposite direction.

• {$m_{n+1}(x)=[\frac{\alpha -\beta}{\alpha}x + \frac{\alpha + \beta}{\alpha}n + c]m_n(x) - \frac{\beta}{\alpha}[n(n-1) + nc]m_{n-1}(x)$}
• {$m_{n+1}(x)=\frac{1}{\alpha}[\alpha(n + (x+c)) + \beta(n - x)]m_n(x) - \frac{\beta}{\alpha}[n(n-1) + nc]m_{n-1}(x)$} where I have split up the {$x$} term into two parts.

Kim and Zeng:

Kim and Zeng substitute directly into their original recursion relation {$\alpha=\frac{c}{1-c}=f_A$}, {$u_1=u_4=\frac{c}{1-c}=-\alpha_A$}, {$u_2=u_3=\frac{1}{1-c}=-\beta_A$}

• Kim and Zeng: {$P_{n+1}(x)=[x - (u_3+u_4)n + \alpha\beta)]P_n(x)-[u_1u_2n(n-1) + u_1u_2\beta n]P_{n-1}(x)$}
• Kim and Zeng: {$M_{n+1}(x)=(x-\frac{(1+c)n + c\beta}{1-c})M_n(x)-(\frac{cn(\beta + n - 1)}{(1-c)^2})M_{n-1}(x)$}

This is the monic version of the Meixner polynomials. Now set {$M_n(x)=(\frac{c-1}{c})^nm_n(x)$}. We get

• Kim and Zeng: {$m_{n+1}(x)=\frac{c-1}{c}(x-\frac{(1+c)n + c\beta}{1-c})m_n(x)-\frac{(c-1)^2}{c^2}(\frac{cn(\beta + n - 1)}{(1-c)^2})m_{n-1}(x)$}
• Kim and Zeng: {$m_{n+1}(x)=\frac{1}{c}((c-1)x+(1+c)n + c\beta)m_n(x)-\frac{1}{c}n(\beta + n - 1)m_{n-1}(x)$}

Multiplying by {$c$} gives us Chihara's relation. Note that {$\frac{c-1}{c}=\frac{1}{\alpha_A}$} as in my definition of the Meixner polynomials. Our definitions agree. Note that solving for my variables {$\alpha_A$} and {$\beta_A$} in terms of {$c$} yields {$1=\alpha_A-\beta_A$}. Which is to say, the unit step is set to be {$1$} and nonzero. (This makes {$F_1$} interesting here, for then we have {$0=1$}.) As for the monic polynomial, aside from this constraint that {$1=\alpha_A-\beta_A$}, we have my original notation for the general recurrence relation. Note also that {$1-c\neq 0$} and so {$\beta_A-\alpha_A\neq 0$}, which simply reaffirms the constraint.

Chihara gives the original conditions as {$0<c<1$} and {$\beta >0$}, which in my variables means {$\alpha,\beta>0$}, {$\alpha < \beta$} and {$c>0$}. Chihara extends these conditions to be {$c\neq 0,1$} and {$\beta\neq 0,-1,-2 \dots$} which in my variables means {$\alpha,\beta\neq 0$}, {$\alpha\neq\beta$} and {$c\neq 0,-\alpha\beta, -2\alpha\beta\dots$}

Chihara writes Meixner's generating function as {$\sum_{n=0}^{\infty}m_n(x;\beta,c)\frac{w^n}{n!}=(1-\frac{w}{c})^x(1-w)^{-x-\beta}$}. In my variables, it becomes:

{$$\sum_{n=0}^{\infty}m_n(x;\beta,c)\frac{t^n}{n!}=\frac{(1-\frac{\beta}{\alpha}t)^x}{(1-t)^x(1-t)^{\frac{c}{\alpha\beta}}}$$}

The orthogonality relation for {$0<|c|<1$}, or in my variables, both {$\alpha$} and {$\beta$} are negative, or one is negative and the other positive, with the negative one having greater absolute value.

• Chihara: {$\sum_{k=0}^{\infty}m_n(k;\beta,c)m_p(k;\beta,c)\frac{c^k(\beta)_k}{k!}=(1-c)^{-\beta}c^{-n}n!(\beta)_n\delta_{np}$}
• Andrius: {$\sum_{k=0}^{\infty}m_n(k;c,\frac{\alpha}{\beta})m_p(k;c,\frac{\alpha}{\beta})\frac{(\frac{\alpha}{\beta})^k(c)_k}{k!}=(\frac{\beta}{\beta-\alpha})^c(\frac{\beta}{\alpha})^nn!(c)_n\delta_{np}$} where I assume {$c=\frac{\alpha}{\beta}$} thus {$\alpha > \beta$}, and {$|c|>1$} implies {$|\beta|>|\alpha|$}.

There is an alternative orthogonality relation for {$|c|>1$}, or in my variables, both {$\alpha$} and {$\beta$} are positive, or one is negative and the other positive, with the positive one have greater absolute value.

• Chihara: {$\sum_{k=0}^{\infty}m_n(-k-\beta;\beta,c)m_p(k;\beta,c)\frac{(\beta)_k}{c^k k!}=(\frac{c}{1-c})^{\beta}c^{-n}n!(\beta)_n\delta_{np}$}
• Andrius: {$\sum_{k=0}^{\infty}m_n(-k-c;c,\frac{\alpha}{\beta})m_p(k;c,\frac{\alpha}{\beta})\frac{(c)_k}{(\frac{\alpha}{\beta})^k k!}=(\frac{\alpha}{\beta-\alpha})^c(\frac{\beta}{\alpha})^nn!(c)_n\delta_{np}$}

The generating function yields this explicit representation of the Meixner polynomial:

• Chihara: {$m_n(x;\beta,c)=(-1)^nn!\sum_{k=0}^n\binom{x}{k}\binom{-x-\beta}{n-k}c^{-k}$}
• Andrius: {$m_n(x;c,\frac{\alpha}{\beta})=(-1)^nn!\sum_{k=0}^n\binom{x}{k}\binom{-x-c}{n-k}(\frac{\alpha}{\beta})^{-k}$}

And also this explicit representation:

• Anne de Medicis: {$m_n(x;\beta,c)=\sum_{k=0}\binom{n}{k}(-x)_k(\beta + k)_{n-k}(-1+\frac{1}{c})^k$}
• Andrius: {$m_n(x;c,\frac{\alpha}{\beta})=\sum_{k=0}\binom{n}{k}(-x)_k(c + k)_{n-k}(\frac{\beta-\alpha}{\alpha})^k$}

There is an identity

• Chihara: {$a^nm_n(x;\beta,a)=m_n(-x-\beta;\beta,a^{-1})$}
• Andrius: {$(\frac{\alpha}{\beta})^nm_n(x;c,\frac{\alpha}{\beta})=m_n(-x-c,c,\frac{\beta}{\alpha})$}

These papers explain how to calculate moments of orthogonal polynomials using combinatorics:

• Sylvie Corteel, Jang Soo Kim, Dennis Stanton. Moments of Orthogonal Polynomials and Combinatorics.
• Mattheiu Josuat-Verges, Martin Rubey. Crossings, Motzkin Paths and Moments.

These papers have formulas for the moments {$\mu_n$} of the Meixner polynomials.

• Jiang Zeng. Weighted Derangements and the Linearization Coefficients of Orthogonal Sheffer Polynomials
• Anne de Medicis. The Combinatorics of Meixner Polynomials: Linearization Coefficients
• Dongsu Kim, Jiang Zeng. A Combinatorial Formula for the Linearization Coefficients of General Sheffer Polynomials.

Anne de Medicis uses Chihara's variables.

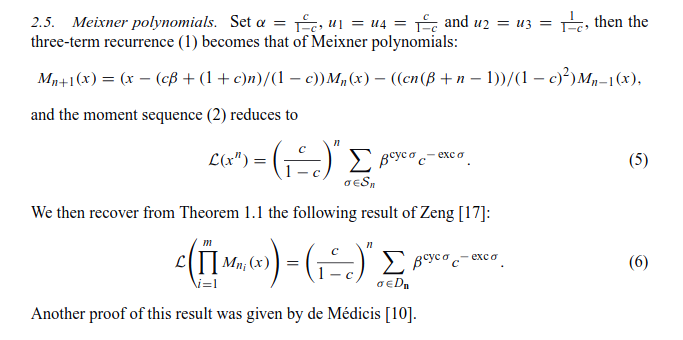
• de Medicis: {$\mu_n(\beta,c)=(1-c)^{-\beta}\sum_{k\geq 0}k^nc^k\frac{(\beta)_k}{k!}$}
• de Medicis: {$\mu_n(\beta,c)=(1-c)^{-n}\sum_{\sigma\in S_n}\beta^{\textrm{cycle}\;\sigma}c^{\textrm{nexc}\;\sigma}$}
• Kim and Zeng: {$\mu_n=(\frac{c}{1-c})^n\sum_{\sigma\in S_n}\frac{\beta^{\textrm{cyc}\;\sigma}}{c^{\textrm{exc}\;\sigma}}$}
• Andrius: {$\mu_n(c,\frac{\alpha}{\beta})=\frac{1}{(1-\frac{\alpha}{\beta})^{c}}\sum_{k\geq 0}k^n(\frac{\alpha}{\beta})^k\frac{(c)_k}{k!}$}

Moments

Kim and Zeng have this general formula for the moments:

{$\mathcal{L}(x^n)=\sum_{\sigma\in S_n} u_1^{\textrm{ad}\;\sigma} u_2^{\textrm{da}\;\sigma} u_3^{\textrm{aa}\;\sigma} u_4^{\textrm{dd}\;\sigma} \alpha^{\textrm{fix}\;\sigma} \beta^{\textrm{cyc}\;\sigma} $}

In my notation

{$\mathcal{L}(x^n)=\sum_{\sigma\in S_n} (-\alpha)^{\textrm{asc}\;\sigma} (-\beta)^{\textrm{desc}\;\sigma} f^{\textrm{fix}\;\sigma} c^{\textrm{cyc}\;\sigma} $}

Distribution

Chihara writes the distribution in his notation as {$j(x)=\left(\frac{d-\rho}{d+\rho}\right)^n\frac{(1+\frac{h}{g})_n}{n!}$} at {$x=\rho n\;\;\;n=0,1,2,\dots$} In my notation this is: {$\omega(x)=\left(\frac{\alpha}{\beta}\right)^n\frac{(c)_n}{n!}$} at {$x=(\alpha-\beta)n\;\;\;n=0,1,2,\dots$}. But this makes sense only if {$\alpha - \beta = 1$} because we need {$t=n$}. Alternatively, we need to replace {$n$} with {$(\alpha - \beta)n$}.

Equivalently, this is: {$\left(\frac{\alpha}{\beta}\right)^n\binom{c+n-1}{n-1}$} if {$(c)_n$} is the rising factorial, as in Chihara's book.

Note that {$f$} does not appear in the distribution. What is the significance of that?

Eulerian number

Eulerian number {$A(n,k)$} is the number of permutations {$\sigma\in S_n$} which have {$k$} ascents, that is, {$\sigma(i)>i$} exactly {$k$} times.

{$A(n,k)=\sum_{i=0}^k(-1)^i\binom{n+1}{i}(k+1-i)^n$}

The Eulerian numbers {$A(n,k)$} and Eulerian polynomial {$A_n(t)=\sum_{k=0}^nA(n,k)t^n$} are linked to the Fubini numbers (the ordered Bell numbers) {$a(n)$} by this formula:

{$a(n)=\sum_{k=0}^{n-1}2^kA(n,k)=A_n(2)$}